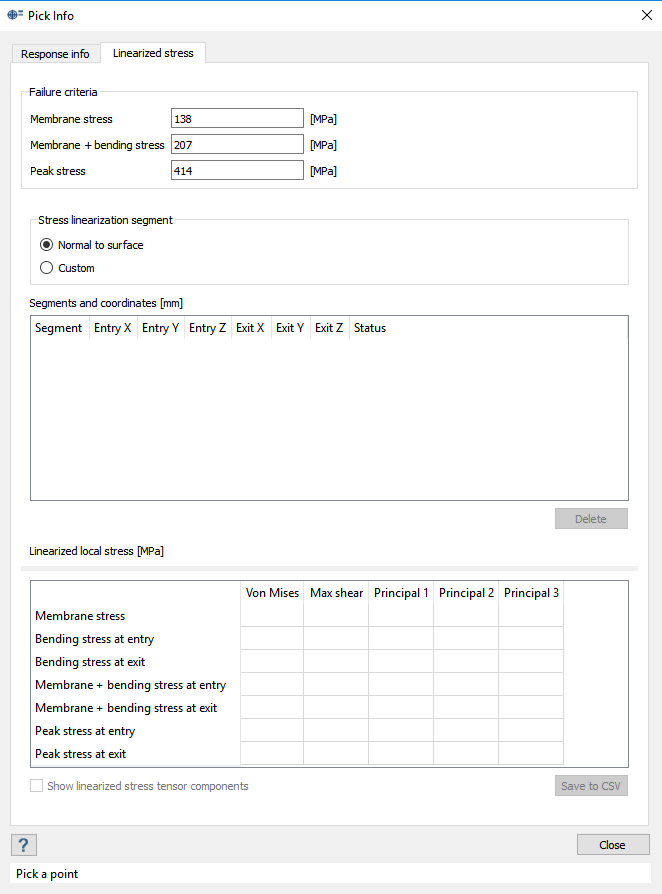
Calculate Linearized Stress
Decompose a through-thickness elastic stress field into equivalent membrane, bending, and peak stresses for comparison with appropriate allowable limits.
Calculation Method
SimSolid method for calculating linearized stress.
Linearized stress decomposes a through-thickness elastic stress field into equivalent membrane, bending, and peak stresses for comparison with appropriate allowable limits.
- The stress is extracted by interpolation in a local coordinate system at all
the points along the line. The local coordinate system is based on the start
and end points of the stress linearization segment. The X-axis of the system is along the segment from entry to exit points. The other two axes are calculated as follows:
- If the local x-axis is not parallel to the global
y-axis:
Zlocal = Xlocal x Yglobal
Ylocal = Zlocal x Xlocal
- If the local x-axis is parallel to the global y-axis:
local y-axis (Ylocal) is negative of global-x if local-x is along positive global-y, and vice versa.
Zlocal = Xlocal x Ylocal
- If the local x-axis is not parallel to the global
y-axis:
- From the extracted stress values above, the average membrane stress tensor +
bending stress tensors at the entry and exit points are calculated using
numerical integration.
σmi=1L∫L/2−L/2σidx
σbiS=−6L2∫L2−L2σixdx
σbiE=−σbiS
σmi = ith component of membrane stress
σi = ith component of extracted stress value
σbiS = ithcomponent of bending stress at the entry
σbiE = ith component of bending stress at the exit
L = Length of the Stress linearization segment
x = position of a point along the segment
- Peak stress and membrane and bending stress are also calculated at the entry
and exit.
σpiS=σiS−(σmi+σbiS)
σpiE=σiE−(σmi+σbiE)
σpiS = ith component of peak stress at the entry
σpiE = ith component of peak stress at the exit
- Finally, invariants for the membrane, membrane + bending, and peak stresses are calculated.