Periodic Boundary Conditions (PBC)

Periodic boundary conditions allow for analysing large, uniformly spaced, repetitive linear and planar structures, for example, frequency selective surfaces (FSS).

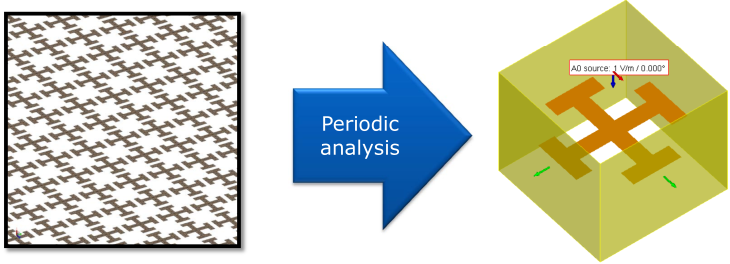
Figure 1. Example of applying PBC to a frequency selective surface, for example a Jerusalem cross.