RD-V: 0110 Friction

The contact friction forces are studied and compared to an analytical solution.



Figure 1.

The contact friction forces are studied between a fixed and moving plate. The friction and reaction forces are used to calculate a displacement which is compared to the applied displacement.

Options and Keywords Used

  • /INTER/TYPE24

Input Files

The following input file is used in this verification problem:

<install_directory>/hwsolvers/demos/radioss/verification/contact/0110_friction

Model Description

The top plate is 254 mm square and 25.4 mm thick. The parts are modeled using solid elements and linear elastic material. The larger bottom fixed plate is model as a rigid by using a rigid body. The bottom plate is fully constrained. The negative y face of the top plate was constrained in the y direction. The coefficient of friction between the plates is 0.05. The top plate has displacements applied to the positive y and z faces by applying imposed velocities using:(1) V = V m 2 [ 1 + sin ( 2 π T t + 3 π 2 ) ] MathType@MTEF@5@5@+= feaagKart1ev2aaatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamOvaiabg2 da9maalaaabaGaamOvamaaBaaaleaacaWGTbaabeaaaOqaaiaaikda aaWaamWaaeaacaaIXaGaey4kaSIaci4CaiaacMgacaGGUbWaaeWaae aadaWcaaqaaiaaikdacqaHapaCaeaacaWGubaaaiaadshacqGHRaWk daWcaaqaaiaaiodacqaHapaCaeaacaaIYaaaaaGaayjkaiaawMcaaa Gaay5waiaaw2faaaaa@4B19@
Table 1. Improved Velocity Inputs
V m MathType@MTEF@5@5@+= feaagKart1ev2aaatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamOvamaaBa aaleaacaWGTbaabeaaaaa@37EF@ T MathType@MTEF@5@5@+= feaagKart1ev2aaatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamivaaaa@36CF@ Total Displacement
565 mm/s 0.009 s Y=2.54 mm
-102 mm/s 0.001 s Z=0.0508 mm

The following system is used: mm, s, Mg.

Simulation Iterations

The contact reaction force was output to the time history file. The reaction force from the imposed velocity applied to the top block’s positive y face were also output to the time history file. The surface to surface /INTER/TYPE24 contact interface was studied.

Results

The displacement applied to the top block’s positive y face was compared to the displacement using:(2) u= L Ewh ( F− Rμ 2 ) MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamyDaiabg2 da9maalaaabaGaamitaaqaaiaadweacaWG3bGaamiAaaaadaqadaqa aiaadAeacqGHsisldaWcaaqaaiaadkfacqaH8oqBaeaacaaIYaaaaa GaayjkaiaawMcaaaaa@4225@
Where,
u MathType@MTEF@5@5@+= feaagKart1ev2aaatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbwvMCKf MBHbqefqvATv2CG4uz3bIuV1wyUbqedmvETj2BSbqefm0B1jxALjhi ov2DaebbnrfifHhDYfgasaacH8srps0lbbf9q8WrFfeuY=Hhbbf9v8 qqaqFr0xc9pk0xbba9q8WqFfea0=yr0RYxir=Jbba9q8aq0=yq=He9 q8qqQ8frFve9Fve9Ff0dmeaacaGacmGadaWaaiqacaabaiaafaaake aacaWG1baaaa@39D1@
Theoretical displacement in the y direction
L MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamitaaaa@36C8@
Length of the top block
w MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamitaaaa@36C8@
Width of the top block
h MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamitaaaa@36C8@
Height of the top block
E MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamitaaaa@36C8@
Young's modulus
F MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamitaaaa@36C8@
Total resultant force at the imposed velocity, calculated from /ANIM/VECT/FREAC
R MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaGaamitaaaa@36C8@
Total contact reaction forces in the z direction from /TH/INTER
μ
Coefficient of friction


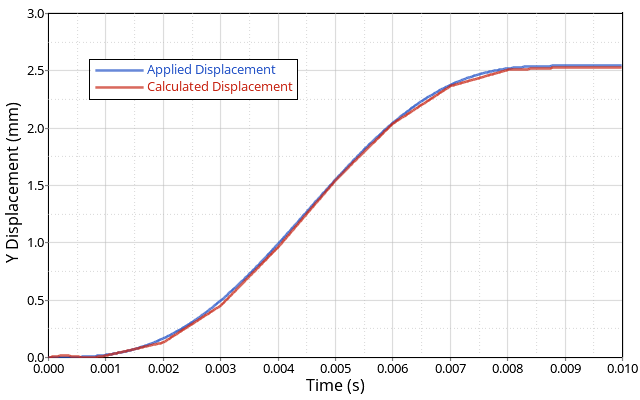
Figure 2. Applied Displacement and the Displacement Calculated

The calculated displacement, where the imposed velocity was applied in the y direction was 2.530 mm. When compared to the applied 2.540 mm displacement, there was a numerical error of 0.39%.

Conclusion

The contact interface friction calculation results in minimal error of 0.39% when compared to theory.

1 Buechler, Miles, Amanda McCarty, Derek Reding, and R. D. Maupin. "Explicit finite element code verification problems." In 22nd SEM International Modal Analysis Conference, Dearborn, MI. 2004